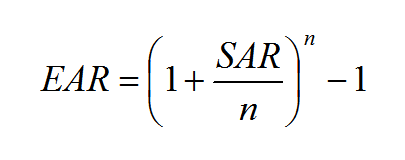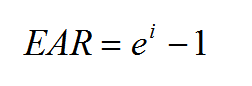A couple of CIPM candidates have contacted me recently asking for an explanation of one of the exhibits in the CIPM Principles Level curriculum that deals with the subjects of stated annual rates and effective annual rates. That exhibit (Reading 4, Exhibit 3) shows a set of “equivalent effective annual rates of return” at different compounding frequencies. Restating the information in that table in words:
- A stated annual rate of 12.0000% is equivalent to an effective annual rate of 12.0000% using annual compounding.
- A stated annual rate of 11.6601% is equivalent to an effective annual rate of 12.0000% using semiannual compounding.
- A stated annual rate of 11.4949% is equivalent to an effective annual rate of 12.0000% using quarterly compounding.
- A stated annual rate of 11.3866% is equivalent to an effective annual rate of 12.0000% using monthly compounding.
- A stated annual rate of 11.3452% is equivalent to an effective annual rate of 12.0000% using weekly compounding.
- A stated annual rate of 11.3346% is equivalent to an effective annual rate of 12.0000% using daily compounding.
- A stated annual rate of 11.3329% is equivalent to an effective annual rate of 12.0000% using continuous compounding.
These statements answer the question of what is the stated annual rate that corresponds to an effective annual rate of 12% at various compounding frequencies (annual, semiannual, quarterly, monthly, weekly, daily, continuous).
(It should be noted that the stated annual rates are rounded at the fourth decimal place.)
For discrete compounding (i.e., when there is a finite number of compounding periods per year), the formula to convert from effective annual rates (EARs) and stated annual rates (SARs) is:
When there is an infinite number of compounding periods involved (i.e., continuous compounding is involved), the formula becomes:
Thus, restating the information in Exhibit 3 with formulae results in the following:
Happy studying!